
During flight training we usually learn turns, turns about a point, S-turns, and the like, in a wide-open area where we have plenty of room to practice. Personally, I learned in the flat plains of North Texas and we had miles and miles of open territory to use. But as you progress in your training and also real-world flying, there are many different situations where the size of your turn matters. Even more specifically, having an understanding of how to even roughly calculate the size of your turns will be useful in a variety of scenarios. So, what is the diameter and radius of standard and non-standard rate turns?
For standard rate (2-minute) turns the diameter is about 1% of airspeed (radius is 0.5%). For non-standard rate turns, the radius and diameter vary based on the given bank angle, rate of turn, and airspeed. As airspeed increases, turn radius increases. As bank angle and rate of turn increase, turn radius decreases.
In the rest of this post we’ll cover the math behind standard rate turn radius, as well as how to calculate desired bank angle, rate of turn, airspeed, or radius for non-standard rate turns. As much as possible we’ll incorporate real-world scenarios so that the concepts are more memorable and applicable.
Radius of Turns
The first thing to remember is that the radius of a turn is a function of the rate of turn and airspeed. Furthermore (and as will be illustrated later), rate of turn is also a function of bank angle and airspeed.
As airspeed increases, radius increases. As rate of turn increases, radius decreases.
You’ll remember that the concept of a standard rate turn is not really about the bank angle, but rather the rate of turn itself – two minutes for 360 degrees, which is three degrees per second.
The bank angle of standard rate turns actually varies with airspeed, but as long as you are flying a standard rate turn it will always take two minutes to make it all 360 degrees of the turn.
Non-standard rate turns on the other hand can have any rate of turn that we want, so calculating the radius starts to become a little more involved. We’ll cover these calculations separately and start with standard rate turns as the math is a bit easier.
Radius of Standard Rate Turns
Standard rate turns are a really helpful mechanism in the air. The concept is simple – regardless of the aircraft or airspeed, the turns will all be the same in that they will take two minutes to complete a full 360 degrees. They are “standard” in that regard.
What varies from aircraft to aircraft and turn to turn is the airspeed at which that turn is made, which then impacts the size of the turn.
Standard rate turns will always take two minutes to complete a full circle, but the faster you are going, the larger that circle will be. Conversely, the slower you are going, the smaller that circle will be.
Turn radius is based on airspeed, bank angle, and rate of turn (which is impacted by both airspeed and bank angle). For standard rate turns, bank angle is already implicitly factored into the rate of turn, which means we only need to use airspeed and rate of turn to calculate the radius.
Chapter 5 of the Pilots Handbook of Aeronautical Knowledge (PHAK) provides some very helpful formulas in regards to calculating all different components of turns including airspeed, bank angle, rate of turn, and radius. For those of us who like math, it’s a fun set of formulas to be able to make all kinds of calculations and nerd out over turn data.
However, I was surprised to find that for very basic standard rate turns there wasn’t a data table for radius. For those of us that want a general rule of thumb for standard rate turns, the PHAK isn’t going to give it to us. So, I built my own.
As a general rule of thumb, the diameter of a standard rate turn is approximately 1% of your ground speed. The radius would be half of that. For example, a standard rate turn flown at 100 knots will yield a diameter of approximately 1 nautical mile and a radius of 0.5 nautical miles.
Using the calculation methods I will present in a moment, I populated the following table with turn radius and diameter data for various ground speeds.
Disclaimer: Do not rely on this data for flight planning purposes. These are general rules of thumb I ascertained from data published in FAA materials. If you are flying in a situation that requires accurate radius and diameter data for the safety of your flight, always consult the operating handbook for the performance data of your particular aircraft and plan accordingly. Do not use the calculations contained in this post or Airplane Academy as a whole as a substitute for proper flight planning required of you as pilot in command.
| Ground Speed (Knots) | Radius (Nautical Miles) | Diameter (Nautical Miles) |
| 60 | 0.3 | 0.6 |
| 70 | 0.4 | 0.7 |
| 80 | 0.4 | 0.8 |
| 90 | 0.5 | 1.0 |
| 100 | 0.5 | 1.1 |
| 120 | 0.6 | 1.3 |
| 140 | 0.7 | 1.5 |
| 160 | 0.8 | 1.7 |
| 180 | 1.0 | 1.9 |
| 200 | 1. 1 | 2.1 |
| 220 | 1.2 | 2.3 |
| 240 | 1.3 | 2.5 |
| 260 | 1.4 | 2.8 |
| 280 | 1.5 | 3.0 |
| 300 | 1.6 | 3.2 |
There are two easy ways of calculating these figures and populating the table: circumference formula and feet per second and rate of turn formula.
Circumference Formula
The first way to calculate the radius of a standard rate turn is to use a circumference formula.
The circumference of a circle is simply the length of the circle if you were to lay it in a flat line. A component of the circumference formula is the radius, so if we know the circumference of a turn, we can solve for the radius.

In other words, a standard rate turn is really a straight-line distance we are flying, it just happens to be curved into a circle. So, we can solve how far we’ll fly during that standard rate turn, factor in our speed, and solve for the radius. Here’s an example.
Let’s say we’re flying at 100 knots and we’ll complete a standard rate turn that, by definition, will take us two minutes to complete. Here is the math and the three steps towards solving for the radius:
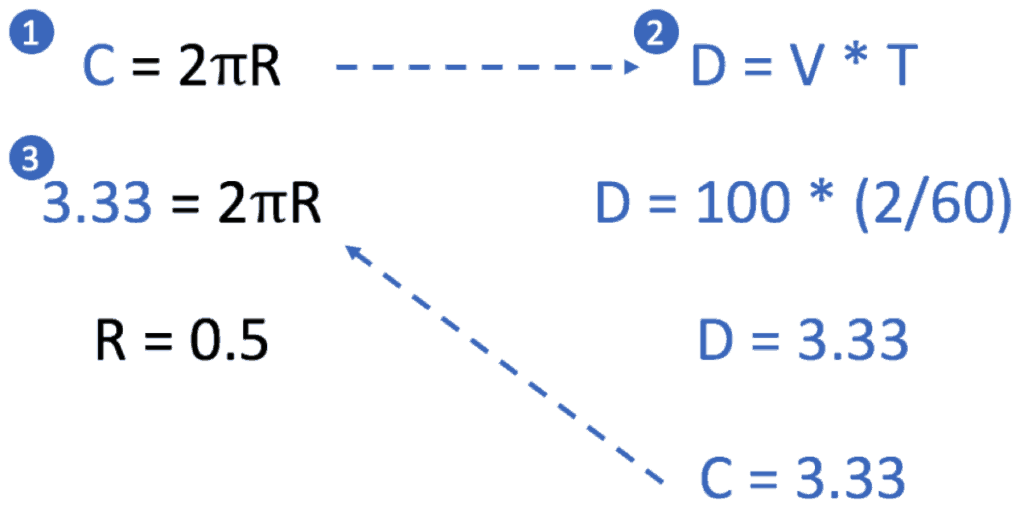
Step 1: The circumference of a circle is really still a distance we’re flying, it just happens to be in a circle. If we can solve for the circumference (distance) we’re flying, we can solve for the radius.
Step 2: The circumference is really a distance we’re flying, and we know that distance is velocity multiplied by time. Knowing this, we can pretty easily solve for distance or circumference. The only trick here is that for time, we know the standard rate turn will take us two minutes, but because knots is presented in knots per hour we need to convert this time figure into hours. You can do this simply by using your two minutes and diving it by 60 to get hours. Plug in those assumptions and the two-minute (standard rate) turn at 100 knots will cover 3.33 nautical miles of distance, which is the circumference of our turn.
Step 3: Simply plug in the 3.33 nautical miles in for circumference and solve for radius by dividing 3.33 by 6.28 (two times pie). Using 3.14 for Pie should work to get you close. (As a side note, while Pie technically has an infinite number of trailing digits after the decimal, NASA uses only the first 15 digits for their telemetry calculations in space. The PHAK uses 4 digits (3.1415).) In our equation, a standard rate turn at 100 knots will have a radius of approximately 0.5 nautical miles. This is based on ground speed and does not factor in wind.
FPS and Rate of Turn Formula
This formula is actually listed in Chapter 5 of the PHAK. For standard rate turns I think this method is overly cumbersome but you will arrive at the same figure as in the above formula. It is more helpful when your rate of turn is something other than standard rate of 3 degrees per second, which we’ll cover in a moment.
Here’s the formula:
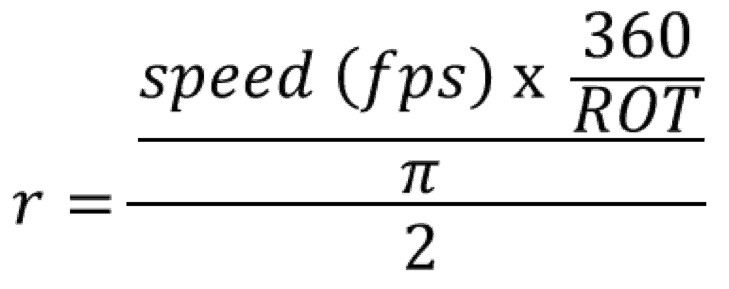
In this formula, rate of turn is simple because a standard rate turn is three degrees per second.
What we do have to calculate is the feet per second given our ground speed. A nautical mile is approximately 6,076.12 feet, which means at 100 knots we will cover 607,612 feet. An hour has 3,600 seconds in, which means 100 knots is about 168.7811 feet per second.
Plug all of this information into the formula and it comes out to about 3,200 feet, which divided by 6,076 feet in a nautical mile comes out to, you guessed it, about 0.5 nautical miles.
Practical Application
As with most learning principles, most of us struggle to remember the lesson unless there is practical application of what we’re learning. Admittedly I didn’t pay much attention to turn radius until my real-world experiences below. There are many ways you can put this information to work for you. I’ll offer up three that quickly come to mind.
Fun
As a learning exercise and interesting flying mission, I recorded the following video to attempt to draw a mathematically perfect “100” in the sky with my flight path. I incorporated the data and calculations above to plan my flight and had a lot of fun with it. Take a look:
Okay, so you might not nerd out for an afternoon like I did to try to draw numbers in the sky. That might not be your idea of “fun”. But there are several other situations that remembering the baseline radius information might be useful for you during flight. I can quickly think of two that have impacted me within the last year.
Missed Approaches
Here is an example of an approach I flew recently that is relevant to our discussion. The missed approach procedure involves making a >180 degree turn to intercept a radial and fly to the published holding procedure.
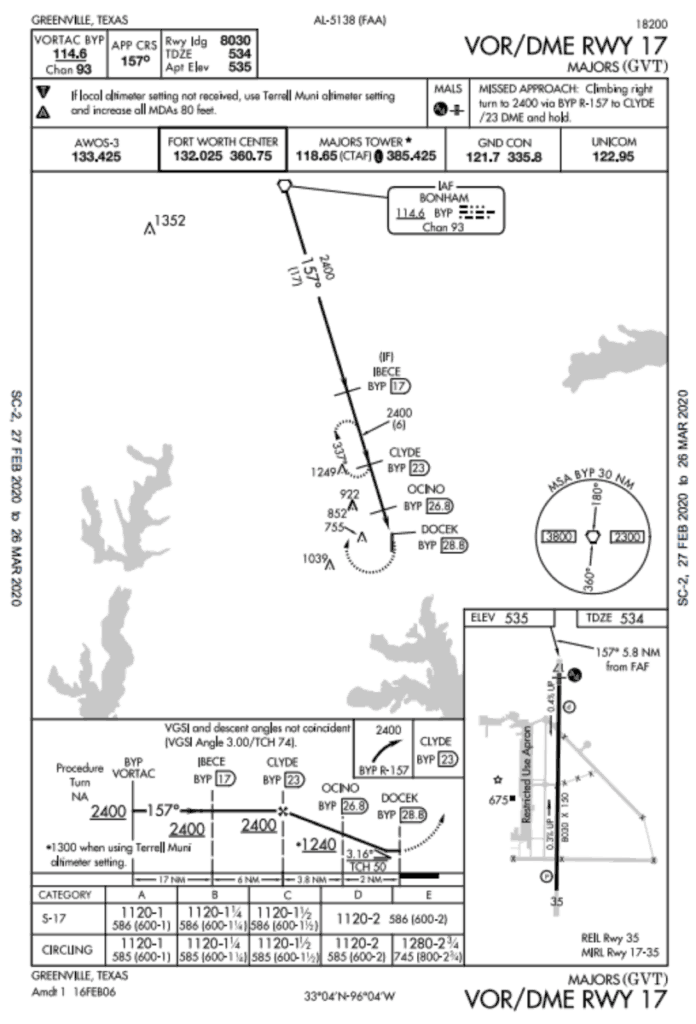
On paper, when you go missed you would plan to fly an intercept angle to join the 157 radial off of the Bonham VOR. Since our heading once we intercept will actually be the reciprocal of that, 337 degrees, I would probably aim to fly a heading of about 010 to make a 30-degree radial intercept just north of the airfield.
But what happens when there’s a strong westerly crosswind? When I’m climbing out on the missed approach at 80 knots, the standard rate turn back to north only has a radius of about 0.4 miles. In other words, I might not make it all the way to the heading of 010 before my flight path intercepts the 157 radial.
It’s not a problem, but I definitely shouldn’t be caught off guard that based on the small radius of the turn coupled with a strong westerly crosswind, there isn’t much ground to cover before I intercept the radial. Keep in mind that these missed approach procedures aren’t always drawn to scale and certainly do not factor in winds.
Mountain Flying
I am based in Texas but in the last few years have fallen in love with the captivating mountain flying that Idaho holds. Flying in Idaho there are many approaches and departures that all take place beneath the mountain tops. You have to fly in and out of valleys into certain airports, and oftentimes make partial or complete turns inside of valleys you can’t immediately out-climb.
In this situation, being confident of your turn radius is paramount. I wasn’t faced with this reality until going up there for the first time and was confronted with an approach that involved a turnaround in a valley to continue the descent into the airfield.
I had plenty of room but that was the first time I really had to trust the radius of a turn and not be intimidated when half way through the turn I was pointed straight at the side of a mountain I couldn’t out-climb. If you jump to the 3:00 mark on the below video you’ll see what I mean:
Mountain flying is a prime example of when you might be flying non-standard rate turns, and need to confidently calculate the radius of those turns. Let’s cover that next.
Radius of Non-Standard Turns
As mentioned above, calculating the radius of non-standard rate turns adds another layer of complexity because rate of turn, the variable that was held constant in standard rate turns at three degrees per second, now varies based on not only bank angle but also airspeed.
The PHAK (mentioned earlier) provides several different formulas for calculating all aspects of turns. You can use these formulas to also solve for desired bank angles, airspeeds, and rate of turn for a desired radius.
The following tables are excerpts from Chapter 5 of the PHAK:
Radius of Turn
One calculation method for radius of turn is if you know the velocity and bank angle:
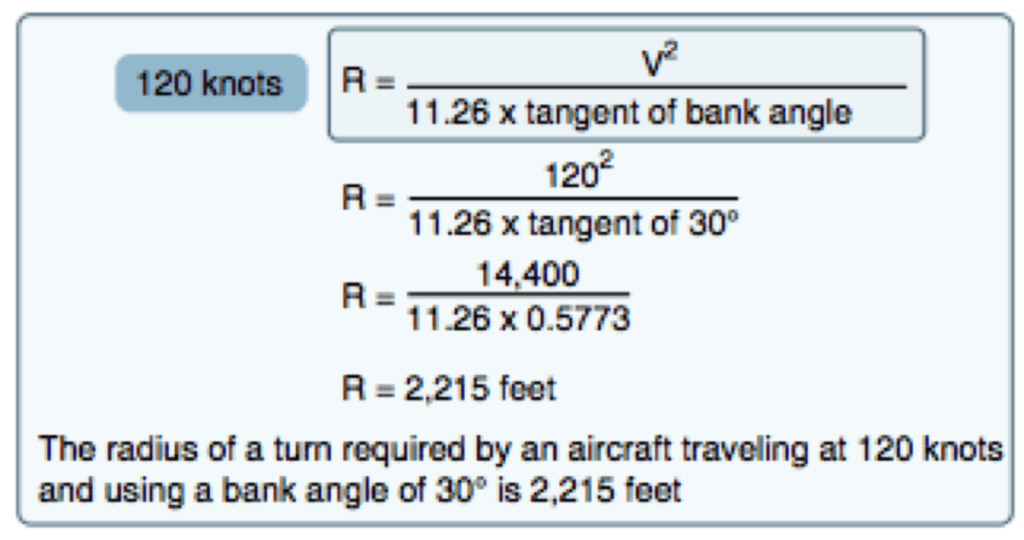
Another calculation for radius is if you know the velocity and rate of turn:
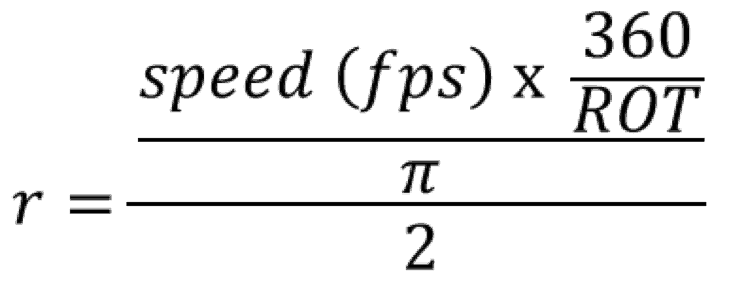
Rate of Turn
To solve for rate of turn, you need to know your bank angle and velocity.
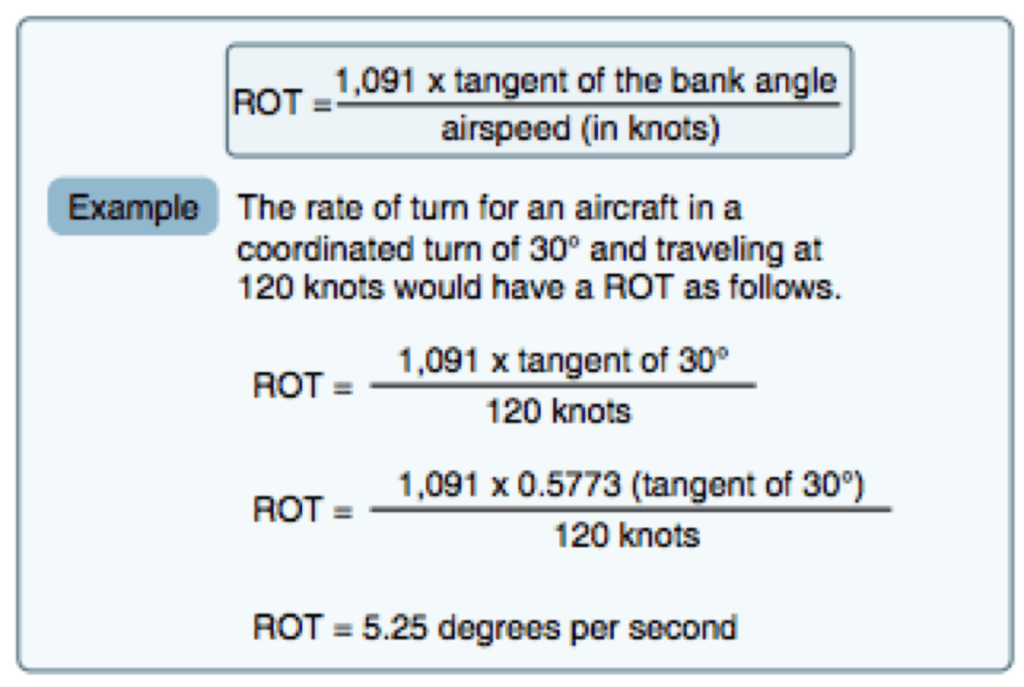
Always remember you can rearrange any of these formulas based on the variables you know and the variables for which you are solving. Here is an example if we know our desired rate of turn (5.25 degrees per second) and airspeed (240 knots) and are solving for bank angle:
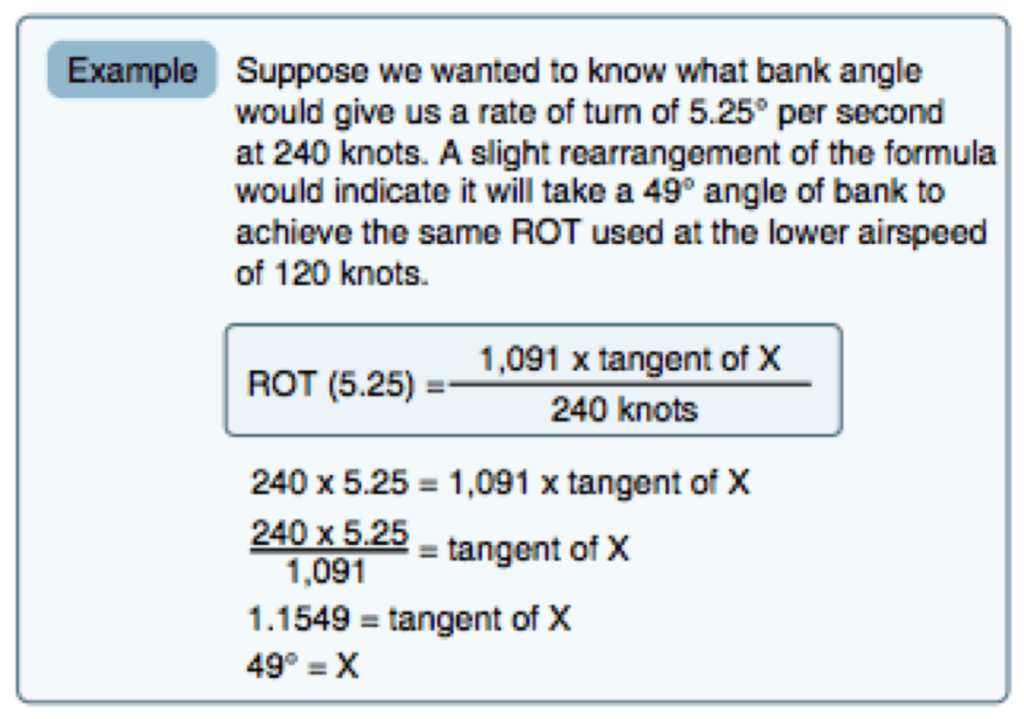
Bank Angle
You can re-arrange any of the above formulas to solve for bank angle (as shown above in Figure 5-58). Alternatively, you can use a guess and check method to plug in assumed bank angles to determine if it gives you the rate of turn or radius you are wanting.
Practical Application
Similar to my mountain flying example and video above, the PHAK provides a real-world scenario of calculating the turning radius for an aircraft accidentally flying into a canyon. It illustrates that the radius of the turn increases airspeed.
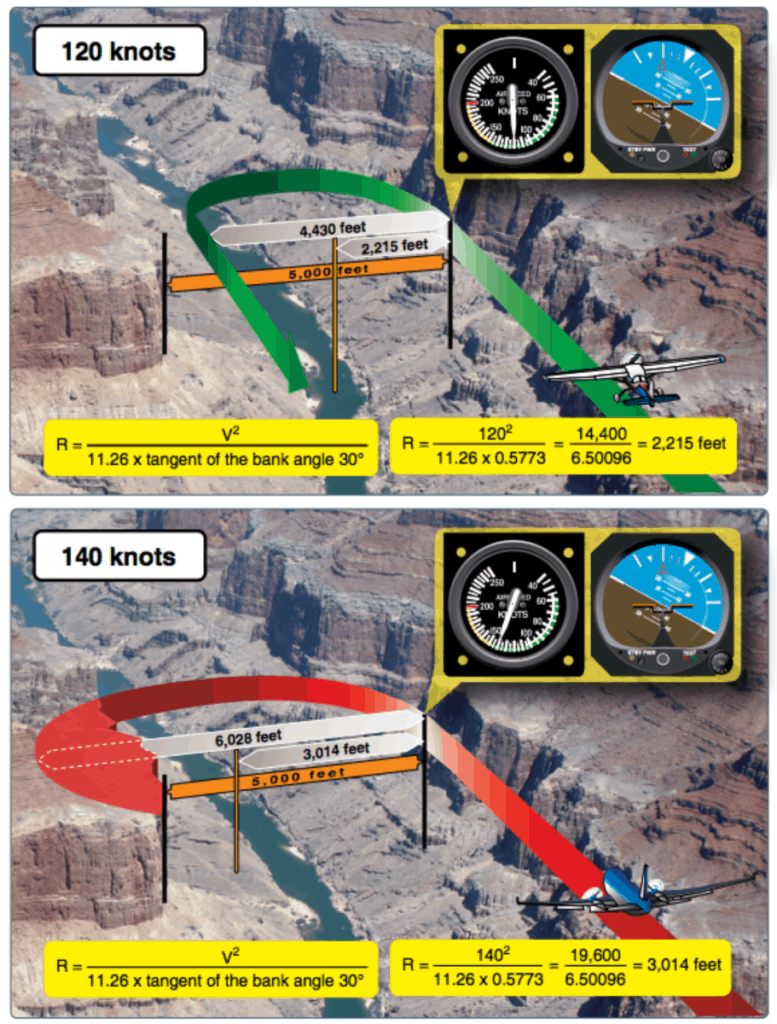
At the same bank angle, the faster aircraft is not able to safely complete the turn in the canyon. Simply increasing the bank angle might not be a viable option either, depending on the airspeed flown as it relates to stall speed in a turn and stress load on the airframe. Often times the best and safest way to decrease the radius of the turn is to simply reduce your airspeed (within appropriate limits).
Conclusion
Familiarizing yourself with the relationship between airspeed, bank angle, rate of turn, and turn radius is not only practical from a fundamental and navigational perspective, but particularly from a safety perspective. While increasing the bank angle of a turn to tighten the radius might not always be an option, oftentimes the easiest way to make tighter turns is to simply slow down.
Either way, be sure to at least have a baseline understanding of the typical turn radius of your aircraft. It will make you a more proficient and safer pilot whether you are in the flat plains of Texas or the beautiful mountains of Idaho.
Have you subscribed to our YouTube channel? You got a taste from the videos above but we are putting a lot of time, resources, and effort into the channel, covering everything from aviation adventures to training tips and FAQs. Subscribe here so you don’t miss out on any new content!



